Stochastic Calculus for Finance 2
Covers Stochastic Calculus for Finance 2 by Steven Shreve
-
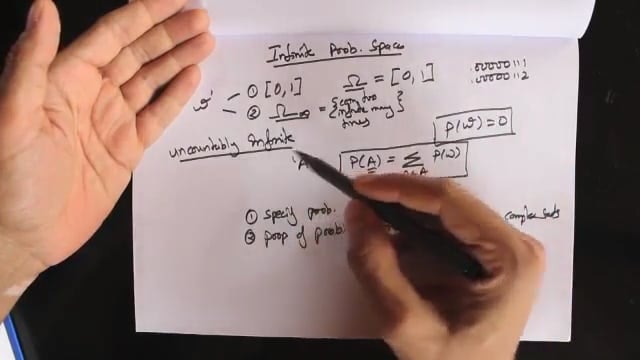
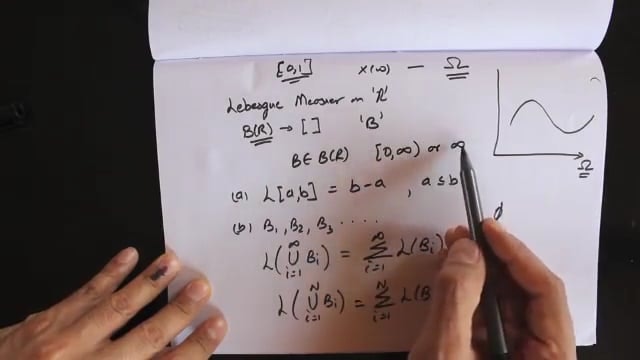
201 - Infinite Probablity Space
Describes Infinite Sample Space, Sigma Algebra, Probability Measure
-
202 - Random Variables and Distributions
Describes random variable and its distribution in an infinite probability space
-
203 (a) - Expectations (Part 1)
Describes Riemann Integral, Lebesgue Integrals and Expectations of a Random Variable.
-
203 (b) - Expectations (Part 2)
Describes how to calculate expected value of a Random variable.
-
204 - Change of Measure (Infinite Probability Space)
Describes the process of change of measure and Radon Nikodym Derivative
-
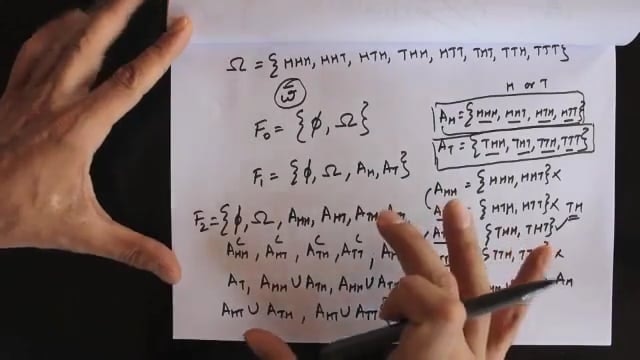
205 - Filteration
Describes Information Modelling and Filteration
-
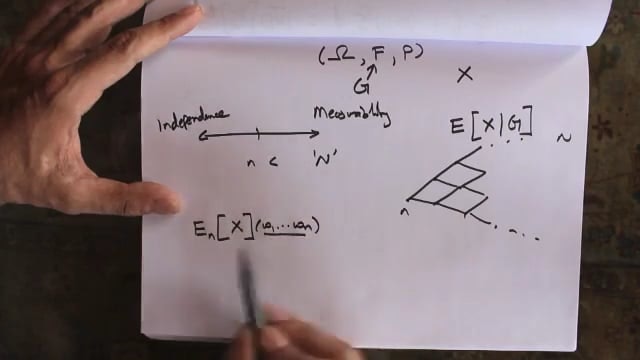
206 - Independence
Describes the meaning of independence of random variables and sigma algebras.
-
207 - Conditional Expectation
Describes conditional expectation under infinite probability space
-
208 - Brownian Motion- Symmetric Random Walk
Describes Symmetric Random Walk and discusses its properties
-
209(a) - Brownian Motion - Scaled Symmetric Random Walk
Describes Scaled Symmetric Random Walk and discusses its properties
-
209(b) - Brownian Motion - Limiting Distribution of Scaled Random Walk
Computes the limiting distribution of scaled random walk
-
209(c) - Brownian Motion - Limiting Distribution of Binomial Model
Computes the limiting distribution of Binomial Model
-
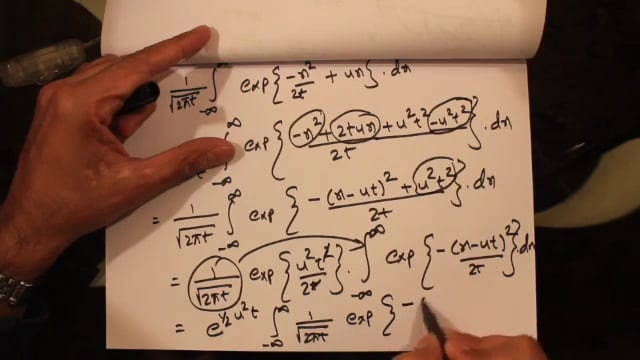
210(a) - Brownian Motion and its Distribution
Defines Brownian Motion and describes its distribution
-
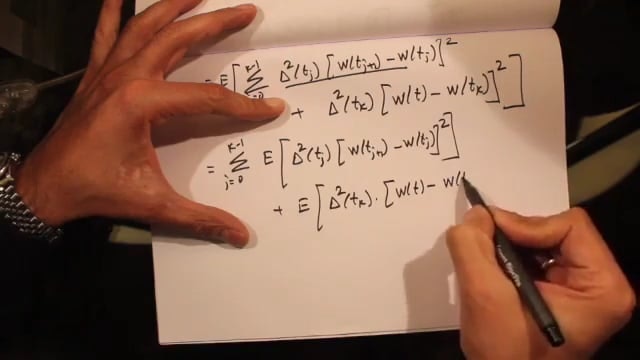
210(b) - Quadratic Variation of Brownian Motion
Describes First Order Variation and Quadratic Variation of Brownian Motion
-
210(c) - Quadratic Variation and Volatility
Calculates Volatility of Geometric Brownian Motion
-
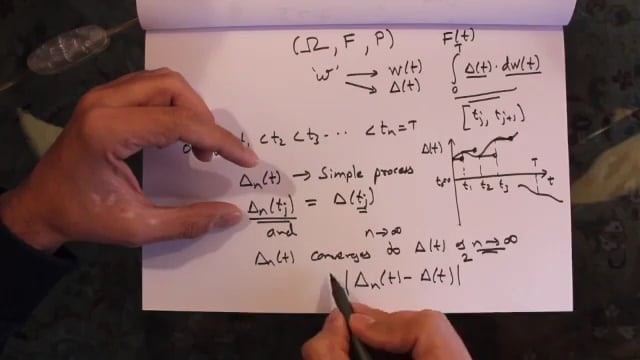
211(a) - Ito's Integral for Simple Integrands (Part 1)
Constructs Ito's Integral for simple Integrand
-
211(b) - Ito's Integral for Simple Integrands (Part 2)
Constructs Ito's Integral for simple Integrand
-
211(c) - Ito's Integral for General Integrands
Constructs Ito's Integral for general Integrand
-
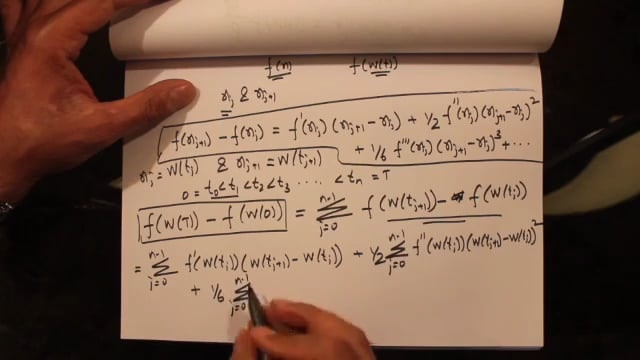
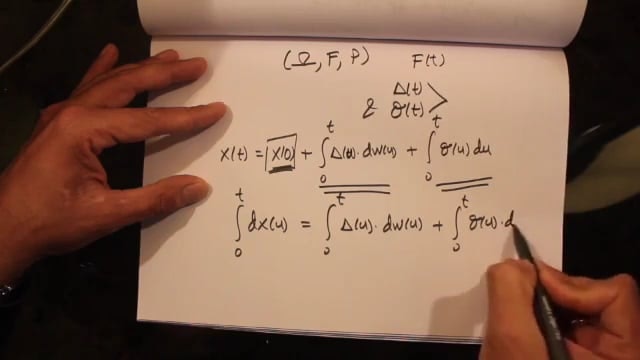
212(a) - Ito's Formula for Brownian Motion
Ito's Formula for Brownian Motion
-
212(b) - Ito's Formula for Ito's Processes
Ito's Formula for Ito's Processes
-
212(c) - Ito's Formula Examples
Examples of how to use Ito's Formula
-
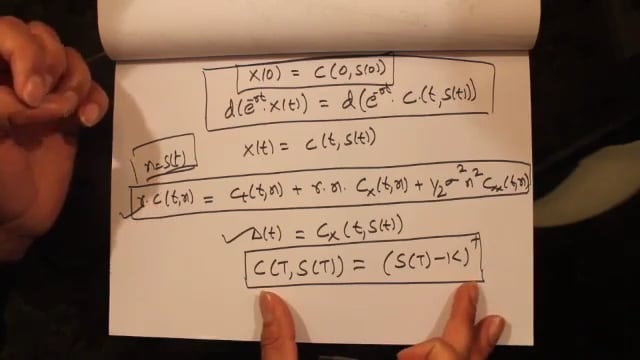
213(a) - Black Scholes Differential Equation
Derives Black Scholes Differential Equation
-
213(b) - Black Scholes Equation - Greeks
Describes greeks of an option and put call parity
-
214 - Multivariate Stochastic Calculus
Stochastic Processes driven by 2 or more Brownian Motion